
Introduction to Factoring Trinomials - reverse of.Anatomy & Physiology, L2 - The Skeletal System.Apologia Biology, Module 13, Phylum Chordata.Multiplying Fractions, cross cancellation.►Found this video at Virtual Nerd: Determining Signs when Factoring a Trinomial In example 4, -xy and -xy will give you -2xy. In example 3, +xy and +xy will give you +2xy. If you use the FOIL method and multiply the Inner and Outer terms (from the binomial factors), you will get either both positive terms or both negative terms to add together, equaling the middle term of the trinomial. Then the sign of the middle term of the trinomial will determine what they both will be.If the last term in the trinomial is + 63, the terms in the binomial factors will either be.If the sign of the last term of a trinomial is positive, the signs between the terms of the binomial factors will either be BOTH positive or BOTH negative. In the second example, the middle term of the trinomial is negative (-2x), showing that the 9 in the binomial factors should be negative, because if you combine -9 and +7, you will get -2.Ģ.In the first example, the middle term of the trinomial is positive (+2x), showing that the 9 in the binomial factors should be positive since it is greater than 7, because if you combine +9 and -7, you will get +2.It is what you use to determine which of the binomial factors will be positive, and which will be negative. So what does the sign of the middle term in the trinomial tell us? So no matter what the middle term is, if the last term in a trinomial is negative, the signs between the terms in the binomial factors will be one positive and one negative.In the second example, both the last term and the middle term were negative.In the first example, only the last term in the trinomial was negative.The signs between the terms in the binomial factors will be one positive and one negative.

If the sign of the last term in a trinomial is negative, such as x² + 2x - 63 To easily determine signs when factoring trinomials:ġ. When these binomial factors are multiplied, they will equal the trinomial.Īfterchecking for a greatest common factor and factoring it out, then you can factor the trinomial. (x + 9)(x - 7) They are called binomial factors. The 2 factors of a trinomial are binomials, and each can be written in parentheses. So with no further delay, check out the next ten trinomial factoring problems and work out the correct " Factoring means turn it into pieces you can multiply."
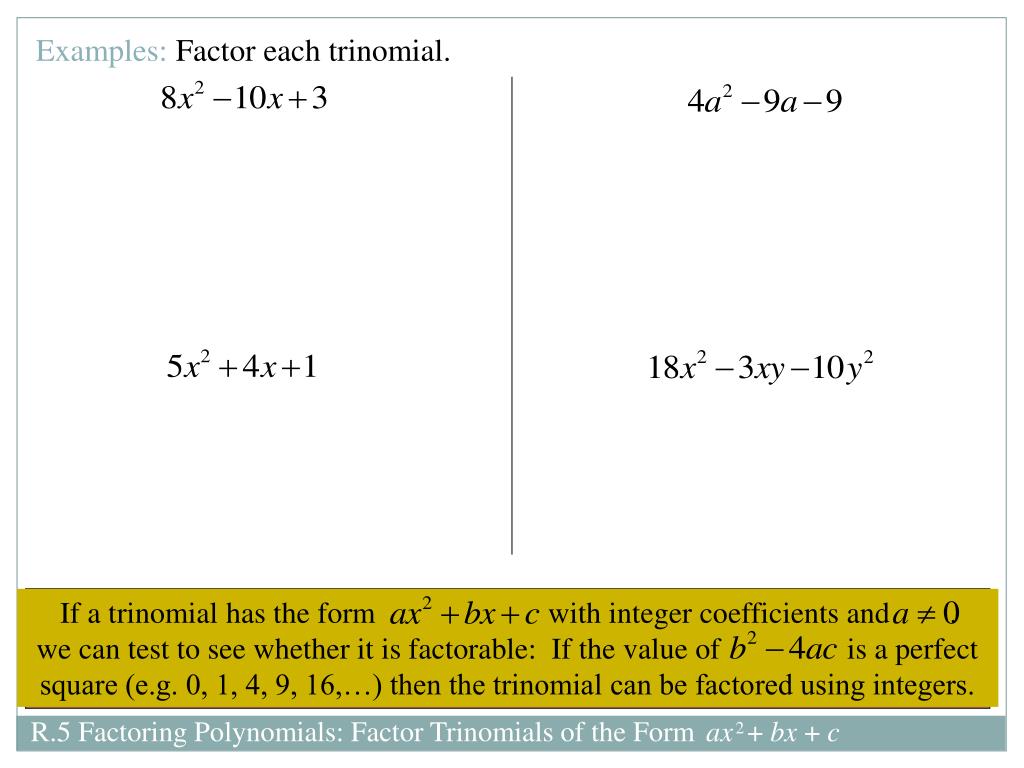
#Factoring trinomials worksheet without middle term how to
If you do not remember how to work the problem, again, please check the prior quizzes as they will detail this out for you. Since the process is the same here as the past quizzes on factoring trinomials, a practice problem will not be presented. If you get the symbol wrong, the entire solution will also be wrong. The process is still the same, you just need to be extra careful in watching your mathematical symbols. In this quiz you will factor trinomials when the first terms may either be greater than the number “1” or lesser than the number “1”. In the first factoring of trinomial quizzes you were give problems where all of the first terms were equal to “1” and the second quiz included numbers greater than the number “1”.

If you have not already taken the quizzes on Factoring Trinomials and Factoring Trinomials, you really should read those introductions first and take the quizzes as they are lead in quizzes to this quiz.


 0 kommentar(er)
0 kommentar(er)
